Some results on Tits' geometries of type 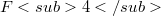
Abstract
It is known that all finite thick geometries of type 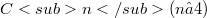 with known parameters are buildings (see [10] and [6]).Several facts suggest the conjecture that the same holds in general.Moreover, a finite thick geometry of type
with known parameters are buildings (see [10] and [6]).Several facts suggest the conjecture that the same holds in general.Moreover, a finite thick geometry of type 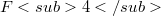 with known parameters is a building unless its parameters are as below (see[6]). It is sensible to conjecture that all finite thick geometries of type
with known parameters is a building unless its parameters are as below (see[6]). It is sensible to conjecture that all finite thick geometries of type 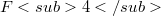 are buildings.I am not able to prove this conjecture.But I collect in this paper some partial results related to this problem.They improve other results given in [9] and [6].
are buildings.I am not able to prove this conjecture.But I collect in this paper some partial results related to this problem.They improve other results given in [9] and [6].
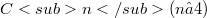 with known parameters are buildings (see [10] and [6]).Several facts suggest the conjecture that the same holds in general.Moreover, a finite thick geometry of type
with known parameters are buildings (see [10] and [6]).Several facts suggest the conjecture that the same holds in general.Moreover, a finite thick geometry of type 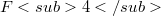 with known parameters is a building unless its parameters are as below (see[6]). It is sensible to conjecture that all finite thick geometries of type
with known parameters is a building unless its parameters are as below (see[6]). It is sensible to conjecture that all finite thick geometries of type 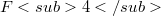 are buildings.I am not able to prove this conjecture.But I collect in this paper some partial results related to this problem.They improve other results given in [9] and [6].
are buildings.I am not able to prove this conjecture.But I collect in this paper some partial results related to this problem.They improve other results given in [9] and [6].DOI Code:
10.1285/i15900932v5n1p51
Full Text: PDF


