Aruler and segment-transporter constructive axiomatization of plane hyperbolic geometry

doi:10.1285/i15900932v22n1p1

Aruler and segment-transporter constructive axiomatization of plane hyperbolic geometry

Victoria Klawitter

Abstract

We formulate a universal axiom system for plane hyperbolic geometry in a first-order language with one sort of individual variables, points (lower-case), containing three individual constants, 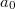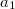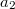$a_0$ , $a_1$ , $a_2$ , standing for three non-collinear points, with 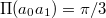$\Pi(a_0a_1)=\pi/3$ , one quaternary operation symbol 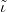$\tilde{\iota}$ , with 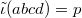$\tilde{\iota}(abcd)=p$ to be interpreted as ` $p$ is the point of intersection of lines $\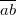overline{ab}$ and $\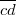overline{cd}$ , provided that lines 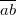$\overline{ab}$ and $\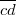overline{cd}$ are distinct and have a point of intersection, an arbitrary point, otherwise', and two ternary operation symbols, 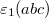$\varepsilon_1(abc)$ and $\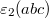varepsilon_2(abc)$ , with $\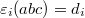varepsilon_{i}(abc)=d_i$ (for $i=1,2)$ 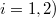to be interpreted as ` 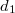$d_1$ and $d_2$ 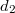are two distinct points on line 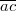$\overline{ac}$ such that 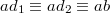$ad_1 \equiv ad_2 \equiv ab$ , provided that 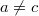$a\not=c$ , an arbitrary point, otherwise'.